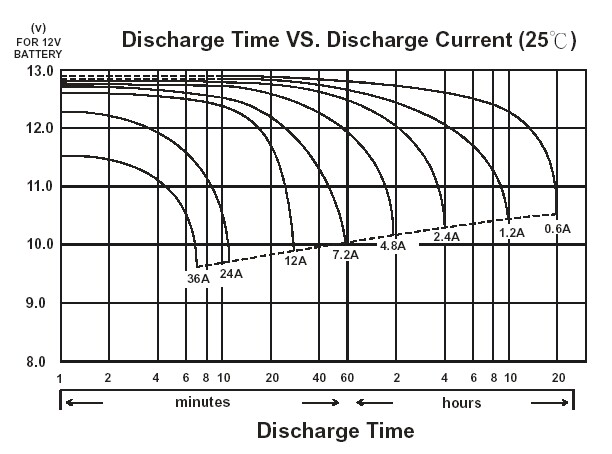
A Simple Method for Discharge Testing Sealed Lead Acid Batteries

Note: the following experiment involves batteries that can be shorted and light bulbs that can get hot.  Use caution if you try this at home!

I recently ordered a pair of 12V 12Ah sealed lead-acid batteries and wasn’t getting the range I expected. Was it my connections or some other problem, or did I just get some weak or poorly made SLAs?

To find out, I ran my own discharge test.  Almost every battery manufacturer publishes discharge curves on their product.  Here is a sample:

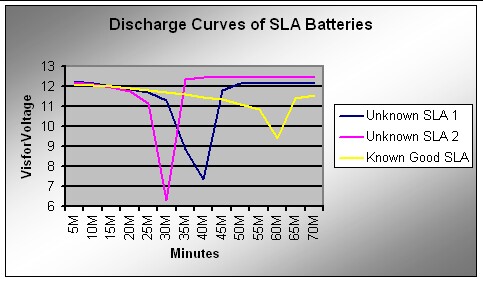
I wanted to choose a load that would discharge the battery in a reasonable period of time, yet give me enough data points for my test.  Looking at the curves above,  It can be seen that a load of about 7.2A should discharge the SLA in about one hour. My definition of “discharged” was where the voltage started to drop off suddenly and headed south of 11V.

A  1 ohm power resistor would draw 12 amps (I=E/R) but it would also have to dissipate 144 watts.  I didn’t have one, and I’m sure it would get extremely hot anyway. Then I got the bright idea to use 12 volt automotive lamps for my load.  They would dissipate the heat well, are rated between 35 and 60 watts or more, and could be combined to add up to most any load I wanted. One other nice feature of using a filament as a load (at least in theory), is as the voltage drops, the resistance of the filament decreases and keeps the current drain more or less constant.

Since they were readily available, I chose my car’s fog lights for the load.  Two 55W fog lamps in parallel = 110 watts.  I (amps) = P (watts) / E (volts) so 110 watts / 12 V = 9.2A.  I charged one of my new SLAs and took it off the charger for an hour to allow it to come to a “resting” voltage. Then, using an inexpensive digital voltmeter I recorded the resting voltage, hooked the battery to my fog lamps and started my stop-watch.  I took one reading at 1 minute, then 5 minutes, and at five minute intervals after that.

I repeated the tests for the other battery as well as another new, but known good 12Ah SLA. My suspicions were confirmed when the suspect pair failed after 25-30 minutes, compared with 55 minutes for the good one.  I entered the data in Microsoft Excel and generated a graph with all three curves on it.

The upswings after the dips represent the battery voltages recovering after I removed the load. Next I plan to run discharge tests on some older batteries I have to see what condition they are in.  The only downside is that it takes up to an hour to test each battery and every five minutes you have to stop what you are doing and read the voltmeter.

What Next?

If you have the money to spend, there are some sophisticated battery chargers that also perform discharge testing.

I considered buying an inexpensive “shunt” that could be put in series with my load. By measuring the voltage across the shunt, I would know the current drain precisely.

There are digital voltmeter interfaces to personal computers you can make or buy. This would allow me to run the test unattended.  It could even shut off the load when the voltage went below a certain threshold.

I can envision a box in which I mounted a set of automotive headlamps that I could switch in and out in different combinations to achieve the desired drain for various battery sizes.

Final note: Even though you could create loads to test SLAs in series, I wouldn’t recommend it. It might be difficult to tell which battery was contributing to an early voltage drop.

© S. Duncan 02/2003

[Home] [E-Bikes & Scooters] [My E-bikes] [Scooterama] [Battery Tech] [LEV Info] [I'm Car Crazy] [Advice & Opinion] [My Photography] [Tendon Pain?] [About Me]